TriTriadic Scales with Seven Tones

(Assembled from the three original tritriadic papers in Xenharmonikon IX, X & XII).

With the exception of pentatonics, seven tone scales are the most widespread type in world music. Whether this near universality is due to psychological preferences (1) or to innate structural superiority is as yet undecided, but heptatonic scales are attested from the 2nd millennium B.C. in the Near East (2).

Since the time of Rameau, it has been recognized that scales of the major or minor type may be partitioned into three triads on roots separated by a perfect fifth (3). A. J. Ellis extended this approach and derived the tonal modes of European music from the circular permutations of scales with mixed triads (4). In 1982, David Lewin generalized the concept to include all scales generable from three identical triads on three equally spaced roots under the name of Riemann Systems (5), which consist of a tonic (T), a dominant (D) and a mediant (M). In independent investigations, these ideas were applied to scales with more than seven tones in non-twelve tone equal temperaments by Chalmers (6) (7) and to seven tone scales constructed from non-major or minor triads by Chalmers and by Ervin Wilson, who suggested the term tritriadic as a replacement for Ellis’s somewhat ambiguous “trichordal.”

Recently, Max V. Mathews and his collaborators have been studying scales generated by triads such as 5:7:9 and 3:5:7 as well as more complex structures (8). Preliminary psychoacoustic results by Linda Roberts (9) (10) indicate that indeed such triads are perceptible musical entities with well-defined harmonic tendencies. These theoretical and experimental activities are an indication of a renewed interest in non-traditional melodic and harmonic musical possibilities by composers.

Although Lewin explicitly recognized the generality of his approach, he limited its application to the 26 “irredundant” seven tone Riemann Systems found in twelve-tone equal temperament and the occurrence of some of them in the standard repertory (5). Mathews, Roberts and Pierce have explored several specific cases of tritriadic scales in Just intonation, but at present seem to be directing their research into other and perhaps more complex areas (8). This paper will focus on the melodic properties of seven tone tritriadic scales as a function of the relative sizes of their dominant and mediant intervals. Among the properties to be examined are Rothenberg’s propriety function (11), which is equivalent to Balzano’s coherence (12), and the existence of (strictly) proper scales in just intonation.

In accordance with Lewin, tritriadic scales may be described by a dominant (D) and a mediant (M) above an arbitrary tonic (T), defined as 0 cents in equal temperament or 1/1 in just intonation. Tritriadic matrices are constructed from T, D, and M in analogy with the fundamental harmonization of the major and minor scales.

	EQUAL TEMPERAMENT			JUST INTONATION
SUBDDOMINANT	-D	M-D	T	2/D	M/D	2/1
TONIC	0	M	D	1/1	M	D
DOMINANT	D	D+M	2D	D	DxM	D2

The above scalar matrices are modulo 1200 or 2/1. In the case of the major scale the tonic chord is 0 400 700 or 4:5:6 (1/1 5/4 3/2).

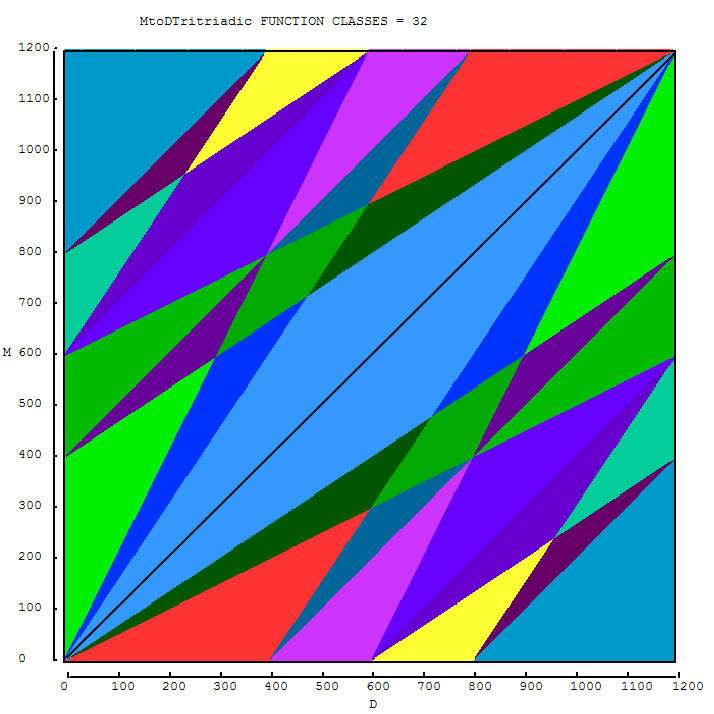
If the magnitudes of D and M are allowed to vary over the range from 0 to 1200 cents, the tonal space DxM will be divided into 32 regions, which correspond to the 32 possible types of tritriadic scale with identical triads. As may be seen in Figure 1-1., the scales consist of 16 classes related by inversion. For example, type III-2, which includes the major and minor modes, is inversionally paired with type II-3, which contains the same intervals in reverse order, i.e., the major scale (T, D, M) is paired with its inverted type (T, -D, -M). This scale has been variously known as the Greek Dorian, Blainville’s “Third Mode” or Von Oettingen’s “Phonic System.” The restrictions on the sizes of D and M which define the various scalar classes are the following, paraphrased from Lewin (5):

D< >      0, 400, 600, 800 or 1200 cents.
M< >     0, ±D, ±2D, +3D modulo 1200 cents.

These conditions are sufficient to partition D into four domains:

I         0 < D < 400    cents
II          400 < D < 600    cents
III         600 < D < 800    cents
IV         800 < D < 1200  cents,

which define the four major types. Classes and subclasses are determined by the size of M in relation to the positions of D, 2D, and -D. The complete listing of the 32 classes is given in Tables 1-1, 1-2. 1-3. and 1-4. Included in these Tables are the defining inequalities which specify the precise relationship between D and M in each class. In order to save space, scales numbered 19 and 26-31 in Tables 1-3. and 1-4. have M-D in place of 1200-D+M even though M is less than D.

Only the Prime forms are shown in Tables 1-1.1-4. and Figure 1-1. The other members of Lewin’s “serial type group” are present, but not explicitly so. The conjugates (T, D, M-D) are in the same major region as their Prime forms, while the inverted (T, -D, -M) and retrograde (T, -D, M-D) forms are among the inversionally related types. For example, both the major and minor modes map into region III-2, but the conjugate of type III-1 is of type II I-3.Although each of these scales comprises three acoustically identical triads, these triads do not necessarily consist of alternate scale degrees or even of melodically equivalent intervals. In fact, only type III-2 scales have tertian chords, and only six of the 32 types have triads of melodically identical form. These consistent types and their triads expressed as ascending scalar intervals (0-M, M-D) are given below:

TYPE     TRIADIC INTERVALS

I-1        seconds

III-2      thirds

IV-2B    fourths

I-3C      fifths

II-3       sixths

IV-4      sevenths.

The inversional pairing is obvious here too. Type III-2 is harmonized with triads built of thirds, while its inverse, II-3 employs sixths.

All the 26 other types have triads whose component intervals, though acoustically identical, span different numbers of scale degrees. This result suggested that an examination of the propriety (11) or coherence (12) might be informative. It was discovered by solving the requisite inequalities in terms of D and M that only 16 types contained proper or strictly proper tunings. In six cases, the only proper solutions were points corresponding to modes of the scale, 0 1 2 3 4 5 6 in 8-tone equal temperament. Four other types had proper solution spaces which consisted of lines. These data are tabulated in Table 1-5. The six types with consistent triads alone had strictly proper solutions, which appear as the interiors of polygonal subregions within the regions of Figure 1-1. Only these types have (strictly) proper solutions in just intonation. The final mode of the seven tone out of eight scale above occurs as an extremal point of one of these regions. The inequ alities defining these regions of propriety are given in Table 6. Of the total DxM space, only about 3% is covered by proper tunings, indicating that such scales are rather rare. The 26 irredundant Riemann Systems in 12-tone equal temperament described by Lewin include only three proper scales, (0, 2, 7), (0, 5, 8), (0, 5, 9), (I-3C, II-3, II-3), and these are all modes of each other.

The set of 32 types of tritriadics was mapped into the equal temperaments which have from seven to twenty-four tones per octave. Fourteen-tone equal temperament was the first to contain all 32 types, and types l-3C and IV-2B were the most frequent.Since the six consistent types would appear to be the most interesting, they were investigated in more detail. Tables 1-7., 1-8., and 1-9. show the frequency of occurrence of these classes in the small-numbered temperaments and the number of improper (I), proper (P), and strictly proper (S) scales of each type. Mixed scales analogous to Ellis’s “principal trichordals” in which the mediants may differ between triads without violating scalar order were also examined, but proved to be too numerous for a preliminary report such as this. As such scales are a feature of tonal music, i.e., the harmonic and melodic minor modes, this may be a fruitful area for further research.

As stated before, the six consistent types are the only ones with proper solutions in just intonation. While improper scales are used in many musical cultures, including our own (Hungarian minor), they require different treatment from the proper scales which are more familiar to western musicians. Two types of tritriadics in just intonation were studied. The first are those scales in which D is a perfect fifth (3/2, 701.9550 cents) and M is a third. Such scales may also be construed as tetrachordal genera where the middle interval of the tetrachord is 9/8. These scales are all of type III-2 and are proper when 305.87<D<396.09 cents. The most consonant of these scales are given in Table 1-10. with their triads in the order T, M, and D. Two examples of scales with mediants other than thirds are also given.

In view of the low abundance of proper solutions in the theoretical study above, it is not surprising that the majority of perfect fifth tritriadics are improper. Most tritriadics are improper, and this remains true even when the functions of the triadic components are interchanged. For example,  the triad 1:5:3 (T, M, D) generates a strictly proper scale, but 1:3:5 and 5:3:1 do not. Likewise the retrograde triad 3:5:1 generates the strictly proper inverse minor mode, but 3:1:5 and 5:1:3 yield improper scales. In other words, if a triad generates a proper scale, its retrograde form (T, M, D → D, M, T) will generate its proper, inverse-conjugate related scale, but the other derived triads apparently will not do so.

Table 1-11. lists some representative triads which were discovered to generate proper tritriadic scales. All proper solutions in just intonation are strictly proper, though because of the listener-dependent resolving power of the ear, the contradictory intervals may sound merely ambiguous, i.e., 64:81:96, the Pythagorean major tuning. Nonetheless, it is true that (strictly) proper tritriadics with reasonable consonance are rare. The 5:7:9 scale proposed by Mathews et al. is a strictly proper example of type IV-2B, but his 3:5:7 triad yields improper scales in all of its six functional arrangements. The traditional 4:5:6 major and Mathews’s 5:7:9 are probably optimally consonant examples of this type of scale. Other common triads which produce improper scales are listed in Table 1-12.

The techniques developed in this preliminary study may be easily applied to the analysis of scales with other than three triads. For example, five tone scales with two triads comprise only two major types and ten subtypes. The conditions are that D < > 0, 600, or 1200 cents and that M < > 0, ±D, or 2D modulo 1200 cents. In the case of the nine tone scale with four triads proposed by Balzano (12), there are six major types and 70 subtypes where D < > 0, 300, 400, 600, 800, 900,   or 1200 cents and M < > 0, ±D, ±2D, ±3D or 4D modulo 1200 cents. The extension to five triads is straightforward. D < > 0, 240, 300, 400, 480, 600, 720, 800, 900, 960, or 1200 cents and M < > 0, ±D, ±2D, ±3D, ±4D, or 5D modulo 1200 cents. There are 10 major classes and numerous subtypes.

This investigation has been very materially aided by a set of computer programs written by the author in BASIC on a COMPAQ DESKPROä II IBM compatible computer to generate, classify and analyze tritriadic scales.

LITERATURE CITED

1. Miller, George A. (1956). The Magical Number Seven, Plus or Minus Two: Some Limits on our Capacity for Processing Information. Psych. Rev. 63: 82-96.
2. Duchenne-Guillemin, M. (1969). La Theorie Babylonienne des Metaboles Musicales. Revue De Musicol. 55: 3-11.
3. Rameau, Jean Phillipe. (1725). Nouveau Systeme de Musique Theorique et Pratique. Ballard, Paris.
4. Ellis, Alexander, John. (1885). in Hermann L. F. Helmholtz, “On the Sensations of Tone”, 1877, translated by A.J. Ellis, Dover Publications, NY, 1954.
5. Lewin, David. (1982). A Formal Theory of Generalized Tonal Functions. J. Mus. Theory 26:23-60.
6. Chalmers, Jr., John H. (1979). Polychordal Arrays of MOS Scales. Xenharmonikon 7+8: 11 pages.
7. Chalmers, Jr., John H. (1982). Construction and Harmonization of Microtonal scales in Non-Twelve-Tone Equal Temperaments. Proceedings of the 8th International Computer Music Conference, Venice, Italy, pp. 534-555.
8. .Mathews, Max V., L.A. Roberts, and J.R. Pierce. (19B4). Four New Scales Based on Non-Successive-Integer-Ratio Chords. J. Acoustic. Soc. Amer. Suppl. 1., 75: S10. Abstr. #E6.
9. Roberts, Linda A. and Max V. Mathews. (1984). Intonation Sensitivity for Traditional and Non-Traditional Chords. J. Acoustic Soc. Amer. 75: 952-959.
10. Roberts, Linda A. (1984). Relation of Consonance Theory to Perception of Chords. J. Acoustic Soc. Amer. Suppl. 1 76: S41. Abstr. #R4.
11. Rothenberg, David. (1978). A Model for Pattern Perception with Musical Applications. Part 1: Pitch Structures as Order-Preserving Maps. Math. Systems Theory 11: 199-234.
12. Balzano, Gerald J. (1980). The Group-Theoretic Description of 12-Fold and Microtonal     Pitch Systems. Comp. Mus. J. 4: 66-84.

John H. Chalmers, Jr.

Department of Music^§

TRITRIADIC SCALES WITH SEVEN TONES. PART TWO: DERIVED FORMS AND STRUCTURAL PROPERTIES.

In the first paper of this series, published in Xenharmonikon IX (Chalmers, 1986a), the general concept of tritriadic scales was introduced and placed in historical context. Tritriadic scales are defined as the union of three triads on roots separated by intervals corresponding to the ratio of the extreme to the initial tone of the triad. The three tones of the triad are assigned the role of tonic (T), mediant (M) and dominant (D). Thus the triad 4:5:6 (T:M:D) generates the major mode as the union of the three major triads on the subdominant (4/3), tonic (1/1), and dominant (3/2). As A.J. Ellis showed long ago (Ellis, 1885), tritriadic scales with non-identical triads are also possible, provided, of course, that the interval D/T is the same for all three. The following discussion, however, will be limited to tritriadic scales constructed from identical triads.

If one allows the sizes of the intervals defining D and M to vary freely over both tempered and just values, the tonal space DxM, where 0 £ D £ 1200¢, 0 £ M £ 1200¢, is divided into thirty-two regions. These regions correspond to the thirty-two possible types of tritriadic scale which may be reduced by one half to sixteen pairs of inversionally related scalar forms.These types are shown in Figure 2-1. (reprinted from Chalmers, 19860).

The occurrence of each of these thirty-two types in the low-number equal temperaments was studied and tabulated in the previous article. Of perhaps greater interest was a preliminary analysis of the properties of each type in terms of Rothenberg’s propriety function (Rothenberg, 1978) and a search for strictly proper instances of each of the thirty-two classes. It was discovered that of the thirty-two classes, only six contained strictly proper scales and that these classes were also the only ones whose triads were melodically consistent. These are types 1-1, l-3C, II-3, III-2, IV-2B, and IV- 4. The principal triads of these scales are composed of seconds, fifths, sixths, thirds, fourths and sevenths respectively. These data are summarized in Table 2-13 (1.) together with data on the secondary (mediant and submediant) triads as well.

This paper will focus mainly on the properties of derived forms of triads and the scales they generate within the thirty-two fold classification discussed above. The melodic properties and the existence of strictly proper tunings will be explored in more detail than was possible in the initial paper.

By using the melodic sequences given in Tables 2-1. through 2-4. of Chalmers (1986a), the sizes of the principal and secondary tritads of each of the classes was determined by counting the scalar steps from tone T to M and from M to D for each of the triads of the scale. The inversional symmetry of the set of classes was used as a check against error. For this reason the inversion of each class is listed at the extreme right of each row of Table 2-1. As may be seen in this tabulation, only the six classes listed above contain triads of the same melodic sequence.

These six classes are the only tritriadic scale types to contain strictly proper members, though an additional ten types do have members that are proper, but not strictly so. While both proper and improper scales have equally valid musical applications and many cultures use both, proper scales are more familiar to western musicians. Accordingly, a search program was written to perform a systematic search for triads which generate strictly proper tritriadic scales.

The program, written in BASIC, examined all ordered triples (T, M, D) composed of the odd numbers 1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25 and 27 interpreted as triads (T:M:D). The set was terminated at 27 as relative consonance is a function of small integer relationships and certain reasonably smooth triads were known which involved numbers that large (i.e., 18:22:27). As will be discussed below, each triad has six permutations because each term of the triad may function as tonic, mediant or dominant in constructing scales. Because of certain elementary symmetries, it is necessary to test only three of the six. The set chosen were the Prime, the form resulting from taking the factors in ascending order of size, the form T:D:M derived from the Prime by a D<->M exchange, and the permutation D:T:M by a D<->T operation on the preceding. These three arrangements will be referred to as Orders 1, 2, and 3. in later parts of this paper. Different Orders of a given triad generate very different scales.

After deleting those combinations which according to Lewin’s definitions and theorems do not produce “irredundant” scales with seven distinct tones (Lewin, 1982), and including all three Orders above, 1038 tritriadics were generated end classified. After a few duplicates resulting from the non-primality of some of the larger integers were removed, thirty-nine triads which generate strictly proper tritriadics were found. These are tabulated in Table 2-2. The distribution according to types is I-1,8; l-3C, 7; II- 3, 6; III-2,6; IV-2B,6; and IV-4, 6. Although in the low-number equal temperaments, types l-3C end IV-2B are the most common, these types ere in second place here; a fact probably neither statistically nor theoretically significant. Obviously, generators of strictly proper scales are rare, and many interesting triads yield only improper scales.

In addition to the permutational operations on triads described above, there are other operations which derive new tritriadic generators from pre­existing ones. These are the four operations of Lewin’s·”serial type group” (Lewin, 1982) and are the Prime or Identity, the Conjugate, the Inverse, and the Retrograde or Inverse-Conjugate. The Prime maps the triad onto itself. The Conjugate replaces the M by D/M and, when applied to the 4:5:6 triad, changes major into minor. The Conjugate triad generates the parallel “minor”·of a tritriadic. The Inverse operation converts T:M:D into T:1/M:1/D and yields a tritriadic which is the inversion of the Prime. This operation acts to convert harmonic triads into subharmonic ones in a manner different from that of the Conjugate which preserves the apparent roots. Historically, this operation changes a “Tonic” system into a “Phonic” system with downward-built chords. Lastly, the Retrograde reverses the order of the triadic factors (T:M:D<->D:M:T) and yields the inversion of the Conjugate.

These operations form a finite group of order four and have certain rather interesting properties. The Prime and Retrograde forms generate tritriadics which are cyclic permutations (modes) of each other, and similarly the Conjugate and Inverse are so related. The Prime and Conjugate are not modes in just intonation. The so-called relative minor is merely a transposition of the Conjugate and a notational artifact due to the fusion of comma-separated pitches in 12-tone equal and mean-tone temperaments. The propriety of all four forms is identical, and the two members of a Conjugate-related pair belong to the same type, if the type is one of the six which contain strictly proper members. This is not true of tritriadics in general, and the four forms may fall into as many as four different classes. These relationships are illustrated in Tables 2-3., 2-4. and 2-5. where they are applied to the 4:5:6 triad. In presenting these tables, the subharmonic types, Conjugate and Inverse, were transformed into integers for clarity. Forms of Order 1. of this triad are all strictly proper, but Orders 2. and 3. produce improper scales, despite their being constructed of exclusively of major and minor triads in various inversions on different roots. The transformation of other triads gives similar patterns. Both the Inversion and Retrograde operations and the second and third Order permutations are excellent ways of generating interesting sounding, new musical materials.

While scales of types III-2 and II-3 are familiar to most musicians as modes of the diatonic scale in 12-tone equal temperament, the melodic properties of the other four consistent tritriadic types are less so. The same may be said too of the other classes. The melodic patterns of the six consistent classes have been calculated from the definitions and are presented in Table 6. The conventional symbols, M, N, and S, have been used for the major tones, minor tones and semitones of types III-2 and II-3, but the less familiar intervals of the four other types are indicated with the non-committal A,B, and C. Each of these scale-types contains three sizes of melodic seconds, but the optimum tuning of these scales, except for the III-2 and II-3 is not clear. Types III-3 and II-3 are harmonically and melodically optimized when M, N, and S approach their conventional just or tempered values (Blackwood, 1985), but the ideal intonation for the other for classes is not as readily apparent. One might hazard that 8:9:10 and 5:7:9 (Mathews et al., 1984) are reasonable just intonations for I-1 and IV- 2B. If the intervals B and C of IV-2B are set equal to each other, the scale reduces to the “Pythagorean” tuning where A=9/8 and B=C=256/243, and the generating triad reduces to 9:3:1. This scale, however, is improper and equivalent to a mode or a form of III-2 or II-3. If A is set equal to B or C, a scale composed of intervals of 3√4 / 3√3 and one 9/8 is produced. This scale is similar to certain limiting tunings of Ptolemy’s Equal Diatonic (Chalmers, 1986b) and might be expressed as 5 5 5 5 6 5 5 in 36-tone equal temperament. Types I- 1 and its inverse are even less tractab1e. If A=B then the scale reduces to 5 5 5 6 5 5 5 in 36-tone equal and if A=C or B=C to 3 4 3 4 3 4 3 or 4 3 4 3 3 4 3 in 24. Although both of these are related to the Ptolemaic genus above and to Islamic scales such as those constructed from tetrachords like 88/81 x 9/8 x 12/11 or even √32/√27 x 9/8 x √32/√27, it is fair to say that tetrachordal analysis is not a very informative or productive way to approach scales of these types.

The situation with the remaining tritriadic types is even more complex. Their melodic patterns all have four or five distinct intervals, thus making any cyclic (“Pythagorean”) or tetrachordal interpretation seem rather forced, however ingenious it might be. These patterns are listed in Table 2-7., with the same conventions as Table 2-6. At this time, it would appear best to take them as they are, whether in just intonation or equal temperament, and enjoy their novel intervallic and structural relationships.

Recently a program for the Macintosh^TM computer has been written in Microsoft8 BASIC to take advantage of the four software-defined voices available on this machine. It should also run without significant changes on the AMIGA™ as well, with possibly better sounding timbres. This program constructs tritriadic scales from triads in their twelve derived forms (three Orders x four Operations). It also classifies, analyses, and plays scales in ascending and descending order. The chosen form of the generating triad and a (meta)progression I IV I III VI V I are sounded in four parts with the tone T doubled at the upper octave, in order not to unduly bias the system against the lnverse and Retrograde forms of the triads and their resulting scales. The chords of the progressions are always of two types, a type determined by the form of the generating triad (l, IV, V) and the Conjugate of this type (III, VI). Because of the reversed role, according to some theories, of T and D in subharmonic chords, the program effectively plays the triads of Inverse and Retrograde forms in their second inversions. The (meta) II triad is omitted from the progression as, in general, it is neither of the Prime nor Conjugate type (Lewin, 1982). Finally, the program selects sequences of scalar tones at random from a span of three octaves and plays them four at a time with individual random durations. While not exactly composition, algorithmic or otherwise, it has served to give some idea of the melodic and harmonic resources of many of the tritriadic scales with seven tones.

Literature Cited

Blackwood, Eosley. 1985. The Structure of Recognizable Diatonic Tunings. Princeton University Press. Princeton, N.J.

Chalmers, Jr., John H.. 1986a. “Tritriadic Scales with Seven Tones.” Xenharmonikon I X. Daniel J. Wolf, ed. Middletown CT.

—– 1986b. Divisions of the Tetrachord. (in preparation).

Ellis, A.J.. 1885. in Hermann L.F. Helmholtz. 1877. On the Sensations of Tone, translated and annotated by A.J. Ellis. Dover reprint edition, Dover Publications. New York, N.Y.

Lewin, David. 1982. “A Formal Theory of Generalized Tonal Functions. ” Journal of Music Theory 26:23-60.

Mathews, Max V., Linda A. Roberts, and J.R. Pierce. 1984. “Four New Scales Based on Non-Successive-lnteger-Ratio Chords.” Journal of the Acoustic Society of America. Supplement 1. 75:S10. Abstract ^#E6.

Rothenberg, David. 1987. “A Model for Pattern Perception with Musical Applications. Part 1: Pitch Structures as Order-Preserving Maps.” Mathematical Systems Theory 11: 199-234.

^§This paper was written while the author was a guest of the Department of Music and the Center for Contemporary Music at Mills College during the summer of 1966. I would like to thank Larry Polanksy, Jody Diamond and David Rosenboom for extending the invitation and their assistance in making the summer enjoyable and productive.

TRITRIADIC SCALES WITH SEVEN TONES. PART THREE: THE M->T AN D D->M MATRICES

INTRODUCTION          

Previous papers in Xenharmonikon IX and X have introduced the topic of Tritriadic scales and placed it in historical context (Chalmers, 1986, 1987a). The primary focus of these early communications was the D->T matrix of which the Major Mode of western music is a familiar example. This paper will extend the discussion of the M->T and D->M matrices which was Initiated in a lecture to the Just Intonation Network in 1986 and an article in 1/1 (Chalmers, 1987b).

Trltriadic scales are generated from a triad symbolized as T:M:D where T is the “tonic” and “M” and “D” are a generalized mediant and dominant (Lewin, 1982). These functions may be defined either in Just Intonation as three factors, i.e., 4:5:6, or as intervals in cents, (0, 386, 702). It is convenient to normalize the triad and the resulting scale to the octave 1/1-2/1 or in cents as 0 to 1200. The union of the “Tonic” triad with two identical triads transposed by an intrinsic, structural interval and its inversion constitutes the tonal matrix of the scale. These subsidiary triads function as generalized Dominants and Subdominants, and secondary triads analogous to Mediants and Submediants may also be defined. Thus it is possible to create chord progressions analogous to the basic cadences of simple tonal harmony (Rameau, 1726) and therein lies much of the interest in these scales, for they provide an entry point into the difficult and complex problem of incorporating microtones or other non-12-tone equally tempered intervals into a (quasi- or neo-) tonal idiom (Chalmers, 1979, 1982; Mathews et al., 1984).

The interval of transposition may be either D, M or their difference D/M (D-M), and it Is the choice of this interval which defines the type of tritriadic matrix. It is also possible to permute the order of the defining set so that each of the terms functions as T, M or D. These permutations specify the order of the triad and its resultant matrix. Lewin identified four operations which interrelate tritriadic scales according to whether or not the M and D intervals are replaced by their inversions and M by its D ­complement. These are the Inverse and Conjugate operations which with the Prime (Lewin ‘s “IDENT”) and the Inverse of the Conjugate form a cyclic group. In more traditional terms, the Conjugate is the parallel Major or Minor and the Inverse is the scale that results from building the chords in the downward direction rather than the upwards Table 3-1. lists the principal tritriadic scales to which a second set of thirty-six may be added by reversing the order of the defining sets.

Despite the seeming complexity of having seventy-two scales generated from one triad, there are actually only six distinct forms consisting of three pairs of matrices and their inversions (Figure 3-1). All of the M->T and D->M matrices can be mapped onto various Orders and forms of the D->T by the operations of column and/or row exchange or circular (“modal”) permutation. These scales are not structurally identical, however, they still differ with respect to their tonics and the harmonic functions of their component tones and chords. These relationships between the different tonal matrices are an area which would appear to be important compositionally and which warrants further investigation.

THE M->T MATRICES. On the D x M lattice of Figure 3-1., the Prime and inverse forms of the M->T tonal matrices appear as rotated and reflected versions of the corresponding D->T matrices. This relationship is true irrespective of the Order of the two types as the shape of the matrix on the lattice is independent of the magnitudes of D and M. The Conjugate and Retrograde forms have not been latticed because, although the Conjugate and Retrograde forms of the D->T matrices appear as translated copies of the Inverse and Prime, the Conjugate and Retrograde of the M->T have the same shape as the D->M matrices. The converse is also true and this fact is another example of the deep relationships between all of the tritriadic scales.

As was done with the D->T matrices (Figure 3-2.), all possible M->T matrices have been plotted on the real, modulo 1200, D x M plane (Figure 3-3.). Similarly, there are 32 types of M->T tritriadic matrix, distinguished by the size of D and M. Not surprisingly, these too correspond to rotated and reflected regions of the D->T map. For example, the Prime and Inverse diatonic scales (D->T types III-2 and II-3) correspond to M->T regions #31 and #15. Although the M->T matrices have boundary conditions analogous to those of the D->T, a numbering system simpler than the earlier and rather complex nomenclature has been employed in the succeeding analyses. The melodic and harmonic properties of these scales are tabulated In Tables 3-2., 3-3., 3-4.,3-5., and 3-6.

FIGURE 3-2 FIGURE 3-3 M->T MATRIX

The melodic sequences of the principal M->T matrices are shown in Table 3-2. The order of the tones within the octave depends solely on the magnitude of M and D, and because the permutations of the generating triad (Orders 1, 2, and 3J and the transformation operations (Conjugate, etc.) merely change the sizes of M and D, the scalar order as defined by the interactions or Mand D is different in each of the regions of Figure 3-3. This redistribution of the order of the scalar functions causes the melodic spans of the principal and secondary triads to vary between regions. Table 3-3. shows the scalar interval spans of the Mand D-M intervals of the triads of each of the types of M->T matrix.

As in the case of the D->T matrices, only six of the scales (#I, #4, #15, #17, #20, and #31) have principal triads with consistent spans as measured in scale degrees. These scales have the further property that their secondary triads are the inverse of the primary, i.e., “minor” rather than “major” or vice versa. It is these scales alone that have tunings which are “strictly proper” (Rothenberg, 1978; Chalmers, 1982, 1986). The linear constraints which must be satisfied for strict propriety are given in Table 3-4.Solutions satisfying these inequalities in Just Intonation are rather rare, especially with small numbers, but a search program yielded 42 instances with integers no larger than 27 (Table 3-5.). Forty-two additional scales may be derived from these by reversing the orders of the generating triads.

An examination of the remaining 26 scales showed that, as in the D­>T case, an additional ten matrices have “proper” tunings (Table 6.). Six of these were modes of the scale which Is seven of the eight tones of 8-tone equal temperament, and four were Infinite sets consisting of certain lines in the D x M plane. The remaining 16 scales are always “improper”, as are the majority of all tunings of all 32 of the M->T matrices.

THE D->M MATRICES. The D->M matrices differ far more radically from the D->T than do the M->T as can be seen in Figures 3-1. and 3-4. Despite their strange appearance, they are nevertheless closely related to the previous two types. The Conjugate and Retrograde forms of the M->T and D->M matrices have each other’s shape In the D x M lattice, and rather surprisingly, the various forms of the D->M may be identified with modes of Orders 2. and 3. of the D->T (Table 1.). It may be also noted that the shapes of the D->M matrices in the D x M lattice resemble the D->T matrices in Balzano’s M x D-M lattice (Balzano, 1980).

FIGURE 3-4 D->M MATRIX

There are, as expected, 32 types of D->M matrix distinguished according to the magnitudes of D and M (Figure 3-4.). One half of the D->M matrices are internal rearrangements (Isomers?) or the other half (Table 3-1.). Tables 3-7, 3-8, 3-3-9, 3-10, and 3-11. describe the melodic and harmonic properties of these scales in a manner analogous to the M->T above. Types #4, #8, #16, #20, #24, and #32 contain strictly proper tunings (Table 3-9.). Regions #4 and #20 contain scales analogous to the diatonic tunings of D->T types III-2 and II-3 and M->T #31 and #15. By the use of a search program, forty-two instances of strictly proper D->M tunings and their inversions have been found in 27-Limit Just Intonation (Table 10.). Similarly, a set of ten scales with merely proper members was found (Table 3-11.), and as usual, the majority of tunings are improper.

DISCUSSION   

Further evidence for the close relation or the three types or tritriadic matrix Is given In Table 3-12. Part  A. lists or  the  proportions  or the various sizes of step interval in each class of scale with strictly proper tunings. These data may be used as a crude measure of the differences between the scalar matrices. Part B. shows the actual sequence of steps of each size in an instance of the Prime or Inverse of each type. More precise distance measures and other perceptual parameters may be calculated from these Intervals (Polansky, 1987a; Rothenberg, 1978; Balzano, 1 980). Simi­lar tables may be calculated for the other tunings of the matrices (see Chalmers, 1987a, for the D->T  cases), but the types with strictly proper tunings in Just Intonation would appear to be of generally greatest interest.

An alternative representation of the six basic scalar matrices is shown in Table 3-13. The characteristic shapes of each of the matrices on the D x M lattice of Figure I . may be expressed as six sets of seven three-dimen­sional vectors whose components are the exponents of the normalized D and M factor s defining each tone or the numbers of units, if the axes of  the lat­tice are labelled In cents, degrees or an equal temperament, or similar log­arithmic transform. Thus, the tone 1/ 1 or 0 becomes (0,0,0) where M=0,1,0 and D=1,0,0, and the remaining six notes are derived accordingly. If M=5/ 4 and D=3/ 2, the tones of matrix #I of Table 13 become 1/1 9/8 4/3 3/2 5/3 5/4 and 15/8 when reduced to one octave. The scale is an ordered set in this representation, and scalar order and tonal functions may be readily com­puted from the knowledge of D, M, and the type of tonal matrix..

The advantage of the vectorial representation Is the ease with which the six scalar matrices may be transformed into each other by matrix mul­tiplication and by which “modes” may be obtained by vector addition. Table 3-14 contains six matrices which interconvert the six sets of vectors of Table 3-13. Matrix A is the identity matrix which maps the set into itself. Matrix B is an Inversion operator which converts any scalar matrix into its Inverse. Matrices C, D, E and F transform the major mode into the D->T, M->T and D->scales.

If one assigns values to M and D and plots out the seven circular per­ mutations or “modes” or any or the six basic shapes on the D x M lattice, one sees immediately that the shapes are invariant and merely that the location of the tone labelled I/ I or O has shifted to a different position on the shape. Hence, adding a constant vector which is the difference between the Initial and final position or the tonic to each or the seven will accomplish the per­mutation, which, in fact, appears as a translation on the lattice. For exam­ple, the Conjugate and Retrograde of the D->T tonal matrices are modes of the inverse and Prime, respectively.

It may be noted that this representation is conceptually similar to the “city block” metric discussed by Polansky (1987a). In this abstract tonal space, neither D nor M are yet interpreted as musical intervals. When values for T, M and D are assigned, the scale is instantiated as an actual tuning. Relationships between scales may be conceived either in terms of different sets of T, M and D, different Orders of T, M and D, and different types of tonal matrix, or equivalently, as an abstract tonal space and a set of computational procedures.

T, M, and D themselves can be thought of as points in a N-dimensional space of perhaps 13 dimensions for 27-limit Just Intonation. In this case the 3-dimensional vectors of Table 13 become N-dimensional, as do the operators of Table 3-14. Modulations from one set of T, M and D to others can then be formalized and computed as rotations and translations in this higher dimensional tonal space.

Ellis (1885) proposed mixed arrays of Prime and Conjugate triads to explain the origin of the tonal modes of traditional Wester European music. This principle can be generalized to include arrays composed of Prime and Inverse triads as well. without introducing undue complications, it is possible to construct tonal matrices whose triads are defined by different sets of T, M, and D. In the D->T case, the magnitude of M may be varied within reasonable limits between the triads while preserving the sense of scalar gestalt, provided that the D to T relationship is retained. Ellis gives the specific example of Poole’s “Double Diatonic,” the F mode of a C major scale in which the third of the dominant triad is 7/6 rather than 5/4. In the M->T arrays, he Ds may be similarly varied, and in the D->M, the M of the M/D triad and the D of the D/M are capable of variation. In these cases, the limits on such variation are those which preserve the scalar intervallic order as exemplified in Tables 3-2 and 7.

Chordal arrays larger or smaller than the ones discussed here have been proposed by several authors to harmonize scales containing other than seven tones (Chalmers, 1979, 1982, 1986). Tonal matrices with two to nine chords each with three to six members were constructed from scales generated by cycles of tempered intervals or repeating suboctaval interval sequences. detailed analyses of the properties of the resulting tonal matrices were not presented, however, and this would appear to be an area worthy of further research.

Finally, it is unlikely that most musicians will be satisfied with as sparse harmonic and melodic resources as are available in a single seven tone matrix. While expanded chordal arrays, mixed matrices, and isotonic scale complexes (Chalmers, 1987b) are obvious palliatives, embedding the scales in larger tonal systems to permit modulation is more compatible with contemporary compositional practice. Although large arrays of justly-tuned intervals such as EIlis’s Duodenarium (ElIis, 1885) have been designed and multinote instruments constructed, i.e., Partch (1949), the rapid prolif­eration of small intervals or limited melodic and harmonic utility makes such solutions cumbersome except for electronic instruments whose into­nation is under dynamic control. For these reasons, the use of equally-tem­pered and other “paratactical” tunings even on computer controlled sound generation systems is recommended (Chalmers, 1986; Polansky, 1987b).

LITERATURE CITED

Balzano, Gerald J. 1980. “The Group Theoretic Description of 12-Fold and Mirotonal Pitch Systems.” Computer Music Journal : 66-84.

Chalmers, Jr., John H. 1979. “Polychordal Arrays of MOS Scales.” Xenharmonikon 7+8, John H. Chalmers, Jr. ed, Houston, TX.

1982. “Construction and Harmonization of Microtonal scales in Non­ Twelve-tone Equal Temperaments.” Proceedings or the 8th International Computer Music Conference, Venice, Italy, pp. 534-555.

1986. “Tritriadic Scales with Seven Tones.” Xenharmonikon IX. Daniel J. Wolf, ed. Middletown CT.

1987a. “Trltriadic Scales with Seven Tones. Part Two: Derived Forms and Structural Properties.” Xenharmonikon X. Daniel J. Wolf, ed. Middletown CT.

1987b. “Tritriadic Transformations.” 1/1 3(1): 1-9.

Ellis, Alexander John. 1885. Various appendices to Hermann L.F. Helmholtz. 1877. “On the Sensations of Tone,” translated and annotated by AJ. Ellis. Dover reprint edition, 1954, Dover Publications. New York, N.Y.

Lewin, David. 1982. •A Formal Theory of Generalized Tonal Functions.” Journal of Music Theory 26:23-60.